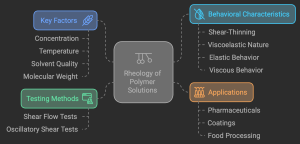
7 Rheological studies of the Polymer Solutions
Muhammad Jawad Ul Rehman
Learning Objectives
Upon completion of this chapter, students will be able to:
- Comprehending Rheological Principles: Students will be able to outline the basic ideas of rheology and discuss how it pertains to the analysis of polymer solution flow and deformation.
- Viscoelastic Behavior of Polymers: Students will be able to identify the differences between elastic and viscous behaviors in polymer solutions and forecast how these characteristics will alter in response to changes in stress frequency, concentration, and shear rate.
- The Function of Polymers in Industry: Students will describe and identify the industrial uses of polymers as gelling agents, thickeners, and stabilizers, and assess how rheological analysis enhances these capabilities.
- Experimental Techniques in Rheology: Students will show that they can characterize the rheological properties of polymer solutions by analyzing and interpreting data from oscillatory shear and shear viscosity tests.
- The students will explain how the behavior of polymer solutions changes from diluted to semi-dilute and concentrated states, as well as how these changes affect flow and viscoelastic properties.
- Impact of External Factors: Students will examine how temperature, solvent quality, and molecular weight affect the rheological behavior of polymer solutions.
- Application of Rheology in Material Design: Students will use their knowledge of rheological characteristics to deduce the molecular interactions and internal structure of polymer solutions, then use this knowledge to suggest material design approaches.

-
Introduction
Rheology, a study of material flow and deformation, is an essential tool for comprehending polymer solutions, where long-chain molecules in solvents display distinctive viscoelastic behavior. Since polymers are commonly used as thickeners, stabilizers, or gelling agents in industries like pharmaceuticals, coatings, and food processing, rheological studies are crucial because they shed light on the internal structure, molecular weight, concentration, and interactions within polymer solutions. Because of the entanglement and interaction of polymer chains, polymer solutions exhibit different rheological characteristics from simple liquids. Depending on the concentration and kind of polymer, polymer solutions can behave both viscously and elastically, in contrast to low-molecular-weight compounds.
Shear flow and oscillatory shear tests are commonly used to investigate rheological behavior in polymer solutions. For example, measurements of shear viscosity show how resistance to flow varies with shear rate. Shear-thinning behavior occurs in many polymer solutions, where viscosity decreases as shear rate increases. The reason for this non-Newtonian behavior is that the polymer chains align along the direction of flow, which lowers intermolecular entanglements and, consequently, flow resistance.
Measuring the storage (elastic) modulus (G’) and loss (viscous) modulus (G”), oscillatory shear tests, in addition to steady shear tests, can enable us to investigate the viscoelastic nature of polymer solutions. This will help us understand how polymer solutions react to stress over time. Elastic behavior (G’) may predominate as frequency rises, particularly in semi-dilute and concentrated solutions, but viscous behavior (G”) frequently predominates at low frequencies. This transition suggests a complex internal structure made up of polymer entanglements and shows that the solution behaves like a solid at high stress frequencies.
One important determinant of the polymer’s rheological characteristics is its concentration in solution. Because polymer chains are widely separated at low concentrations, they behave similar to diluted solutions, in which each chain flows freely. Chains begin to overlap as concentration rises, resulting in semi-dilute solutions where the viscosity is greatly increased by chain interactions and entanglements. Under some circumstances, highly concentrated solutions exhibit solid-like characteristics and behave more like an entangled network.
The rheology of polymer solutions is also affected by temperature, solvent quality, and polymer molecular weight. While poor solvent quality can cause aggregation and increased viscosity, higher temperatures frequently decrease viscosity because they increase the mobility of polymer chains. Chain entanglements are influenced by molecular weight; stronger viscoelastic behavior results from higher molecular weights because of increased chain entanglement.
Explanation of key concepts, theories, and principles
Viscosity and rheology
Rheology is the study of matter when it flows or is deformed, and rheometry is the technique used to examine a material’s rheological behavior. Rheology thus explains forces and deformations over time.
As the field has developed, it is now more concerned with solid deformation and the complex behavior of viscoelastic materials, which can exhibit both liquid and solid characteristics depending on the forces and deformations applied to them.
Rheology generally includes:
- examining how materials react to deformations and applied forces
- investigating intricate flow patterns, particularly in non-Newtonian fluids
- determining the viscoelastic characteristics of materials
- analyzing shear-dependent processes such as shear thickening or thinning
In contrast, viscosity is a particular property in the field of rheology. Viscosity is:
- An indicator of a fluid’s resistance to deformation or flow
- expressed as the shear stress to shear rate ratio.
- Usually stated in units such as centipoise (cP) or Pascal-seconds (Pa·s),
- A crucial element in establishing the “thickness” or fluidity of a fluid
Shear Flow and Shear rate
The rate at which a fluid is “worked” or sheared during flow is known as the shear rate. Technically speaking, it refers to the speed at which fluid layers or laminae pass one another. Both the flow’s geometry and speed affect the shear rate.
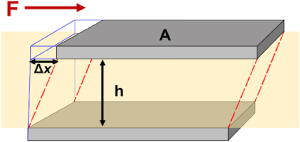
For instance, shear is applied to a sample that is positioned between two plates, with the upper plate acting as the moving plate that will move when shear force, F, is present and the lower plate acting as the stationary plate that will not move. The force (F) acting over a unit area (A) is known as the shear stress (σ), which is the external force. [1]
σ = F/A Eq. (1)
The bottom layer stays constant and parallel to the given height, h, known as the gap, while the top layer moves to a given distance, Δx, in response to this force. We refer to this geometry as plate-plate arrangement or parallel plate. As demonstrated below, Eq. (2) can be used to calculate the displacement gradient across the sample, or shear strain (γ).
γ = Δx/h Eq. (2)
When flow behavior is impossible, the strain for a solid material will be limited by the applied stress. On the other hand, for a liquid material where the constituent components can move relative to one another and create the shear rate, γ˙, which is the rate of change of shear strain with time Eq. (3), the shear strain will continue to increase for the duration of applied stress.
γ˙ = Δγ/Δt Eq. (3)
Viscous/flow behaviors
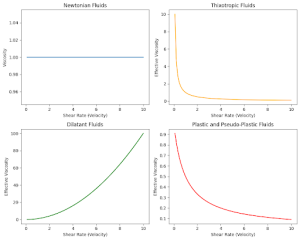
Newtonian (constant viscosity)
Ideally, Newtonian fluids are viscous and follow Newton’s law of viscosity, which states that shear stress and shear rate are linearly related. As a result, viscosity is independent of both shear rate and shear stress
Non-Newtonian (viscosity changes with shear rate)
The viscosity of non-Newtonian fluids exhibits a non-linear dependence on the applied shear rate or shear stress.
Pseudoplastic behavior
Shear thinning or pseudoplastic flow, in which the fluid viscosity decreases as the shear rate increases. Coatings, shower gel, polymer melts, paints, ketchup, and glues are examples of materials that exhibit this behavior frequently. At low shear rates, when the state is at rest, shear thinning fluids exhibit a constant viscosity value or zero shear viscosity plateau, η°. However, as shown in Figure 02, a notable drop in viscosity could be seen at a critical shear rate or shear stress, indicating the start of the shear thinning region.
For instance, disentanglement of polymer chains during flow is the reason for the observed shear thinning of polymer melts and solutions. When at rest, polymers with a high enough molar mass are always entangled and randomly oriented. They start to separate when enough shear is applied, which lowers the viscosity. Shear thinning is referred to as thixotropic if it is time-dependent.
Dilatant behavior
Shear thickening behavior happens when viscosity rises as shear stress or shear rate increases. This phenomenon is known as dilatancy. Shear thickening is referred to as rheopectic if it is time-dependent. Shear thickening is typically seen in dispersions or particulate suspensions that contain a high concentration of solid particles, such as wet sand, ceramic suspensions, and starch dispersions.
Thixotropic behavior
The term “thixotropic behavior” describes a fluid’s tendency to gradually return to its initial viscosity after being exposed to shear stress (such as stirring or agitation); in other words, the fluid becomes less viscous when actively moved and regains its thicker consistency when left undisturbed.
Typical instruments involved in measuring viscosity and rheometric analysis
Viscometer:
A device used to measure a fluid’s viscosity is a viscometer, also known as a viscosimeter.
Rheometers:
Rheometers are devices used to measure the viscosities of liquids that change depending on the flow conditions. Therefore, one could think of a rheometer as a particular kind of viscometer.
A rheometer measures a fluid’s flow behavior under multiple conditions, such as viscosity, elasticity, stress, and strain across a range of shear rates. However, a viscometer only measures a fluid’s viscosity under a single flow condition. This makes the rheometer suitable for complex fluids with non-linear flow properties; in other words, a rheometer provides a deeper understanding of a material’s flow characteristics, whereas a viscometer is more straightforward and only measures viscosity.


Discussion of a peer reviewed paper that relates to your chapter topic
The behavior of shear flows over superhydrophobic surfaces is thoroughly examined in the paper “Modeling of Shear Flows over Superhydrophobic Surfaces: From Newtonian to Non-Newtonian Fluids” [2]. It discusses the advancements and difficulties in modeling Newtonian and non-Newtonian fluids. The local slip length at the liquid/air interface, which is influenced by variables such as flow dynamics and microstructure geometry, is of particular interest. The singularity at the boundary between slip and no-slip areas is also emphasized in the study as a significant obstacle. The study notes that although modeling techniques for Newtonian flows have greatly improved, non-Newtonian flows—in particular, shear-thinning and yield stress fluids—need more research. Understanding non-Newtonian dynamics requires the development of models that accurately depict intricate interactions at the liquid/air interface.
Examining shear flow dynamics, this paper highlights the function of viscosity in both Newtonian and non-Newtonian fluids as well as how it interacts with superhydrophobic surfaces. Established theories assume a flat liquid/air interface with no shear conditions, and viscosity for Newtonian fluids is constant regardless of shear rate. These models form the basis for more intricate methods that include slip conditions and interface deflection. On the other hand, non-Newtonian fluids with variable viscosity that is dependent on shear rate include yield stress fluids and shear-thinning fluids. The study emphasizes the difficulties in simulating these fluids, pointing out that elements such as decreasing viscosity with increasing shear rate complicate their flow dynamics over superhydrophobic surfaces.
By generating slip conditions that affect the effective viscosity of both fluid types, superhydrophobic surfaces play a crucial role. The stick-slip behavior at the liquid/air interface in non-Newtonian fluids can lead to nonlinear effects, which makes modeling even more challenging. The study examines numerical and mathematical models that tackle these intricacies, pointing out important advancements in Newtonian fluid dynamics while recognizing the ongoing development of non-Newtonian research. In the end, it emphasizes how crucial sophisticated modeling methods are to precisely represent the interactions between non-Newtonian fluids and superhydrophobic surfaces in a range of scenarios.
Interactive elements (e.g., quiz, simulation, or video)
Understanding viscosity:
Fluid Mechanics – Viscosity and Shear Strain Rate in 9 Minutes!
Quiz:
References:
[1] Ramli, H., Zainal, N., Hess, M. & Chan, C. (2022). Basic principle and good practices of rheology for polymers for teachers and beginners. Chemistry Teacher International, 4(4), 307-326. https://doi.org/10.1515/cti-2022-0010
[2] Hossein Rahmani, Faïçal Larachi, and Seyed Mohammad Taghavi (2024). Modeling of Shear Flows over Superhydrophobic Surfaces: From Newtonian to Non-Newtonian Fluids. ACS Engineering Au 2024 4 (2), 166-192 https://doi.org/10.1021/acsengineeringau.3c00048
