We said in Section 1.5 that chemists use two models for describing covalent bonds: valence bond theory and molecular orbital theory. Having now seen the valence bond approach, which uses hybrid atomic orbitals to account for geometry and assumes the overlap of atomic orbitals to account for electron sharing, let’s look briefly at the molecular orbital approach to bonding. We’ll return to this topic in Chapters 14, 15, and 30 for a more in-depth discussion.
Molecular orbital (MO) theory describes covalent bond formation as arising from a mathematical combination of atomic orbitals (wave functions) on different atoms to form molecular orbitals, so called because they belong to the entire molecule rather than to an individual atom. Just as an atomic orbital, whether unhybridized or hybridized, describes a region of space around an atom where an electron is likely to be found, so a molecular orbital describes a region of space in a molecule where electrons are most likely to be found.
Like an atomic orbital, a molecular orbital has a specific size, shape, and energy. In the H2 molecule, for example, two singly occupied 1s atomic orbitals combine to form two molecular orbitals. There are two ways for the orbital combination to occur—an additive way and a subtractive way. The additive combination leads to formation of a molecular orbital that is lower in energy and roughly egg-shaped, while the subtractive combination leads to a molecular orbital that is higher in energy and has a node between nuclei (Figure 1.18). Note that the additive combination is a single, egg-shaped, molecular orbital; it is not the same as the two overlapping 1s atomic orbitals of the valence bond description.
Similarly, the subtractive combination is a single molecular orbital with the shape of an elongated dumbbell.
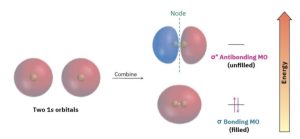
Figure 1.18 Molecular orbitals of H2. Combination of two hydrogen 1s atomic orbitals leads to two H2 molecular orbitals. The lower-energy, bonding MO is filled, and the higher- energy, antibonding MO is unfilled.
The additive combination is lower in energy than the two hydrogen 1s atomic orbitals and is called a bonding MO because electrons in this MO spend most of their time in the region between the two nuclei, thereby bonding the atoms together. The subtractive combination is higher in energy than the two hydrogen 1s orbitals and is called an antibonding MO because any electrons it contains cannot occupy the central region between the nuclei, where there is a node, and thus cannot contribute to bonding. The two nuclei therefore repel each other.
Just as bonding and antibonding σ molecular orbitals result from the head-on combination of two s atomic orbitals in H2, so bonding and antibonding π molecular orbitals result from the sideways combination of two p atomic orbitals in ethylene. As shown in Figure 1.19, the lower-energy, π bonding MO has no node between nuclei and results from the combination of p orbital lobes with the same algebraic sign. The higher-energy, π* antibonding MO has a node between nuclei and results from the combination of lobes with opposite algebraic signs. Only the bonding MO is occupied; the higher-energy, antibonding MO is vacant. We’ll see in Chapters 14, 15, and 30 that molecular orbital theory is particularly useful for describing π bonds in compounds that have more than one double bond.

Figure 1.19 A molecular orbital description of the C–C π bond in ethylene. The lower- energy, π bonding MO results from an additive combination of p orbital lobes with the same algebraic sign and is filled. The higher-energy, π* antibonding MO results from a subtractive combination of p orbital lobes with opposite algebraic signs and is unfilled.

